in a biprism experiment the fringe width is 0.4mm

• Bihar Board
• Online Class
• Ask Doubt on Whatsapp
• Search Doubtnut

In a biprism experiment, fringe width is 0.4 mm when the eypiece is at a distance of 1 mm from the slit. If eyepiece is moved without changing any other arrangment then the change in fringe width is

The correct answer is: a, β 2 = d 2 d 2 β 1 = 0.75 × 0.4 × 10 − 3 1 0.3 m m thus, change in fring width is, β 1 − β 2 = 0.4 − 0.3 = 0.1 m m.

Topper's Solved these Questions

Similar questions.

In a biprism experiment, the fringe width is 1.5mm when the eyepiece is 1.2 m from the salt. What will be the fringe width if the eyepiece is moved towards the slit by 0.2m, with no other change in the experimental setup?

In young's double slit experiment with monochromic light, fringes are obtained on a screen placed at some distance from the plane of slits. If the screen is moved by 5 cm towards the slits, then the change in fringe width is 30 μ m if the distance between the slits is 1mm, then calculate wavelength of the light used.

Knowledge Check

In a biprism experiment the band width is 0.4 mm when the eye piece is at a distance of 1m from the slit. if the eye piece is moved through a distanc eof 25 cm towards the biprism, without changing any other arrangement, then the change in fringe width is, in a biprism experiment, the fringe width is 0.4mm. the distance between the fourthh dark fringe and the sixth bright fringe is, in a biprism experimentn the distancce virtua images of the slit is 2.5 m m and the eypiece is a distance of 1 m from the slits. if the fringe width is 0.3 mm then the frequency of sources of lights is.

In a biprism experiment , fringe width is measured as 0.4 mm . When the eyepiece is moved away from the biprism through 30 cm, the fringe width increase by 50% if the two virtual soruces are 0.6 mm apart, find the wavelength of light used.

In a biprism experiment, the fringe width is 1.4 m m with light of wavelength 6000 Å . What will be the fringe width if light of wavelength 5400 Å is used, with no other change in the experimental setup?

In a biprism experiment, interfernce bands are obtined in the focal of the eyepiece which is at a distance of 1.2 m from the slit. The distance between the two virtual images of the slits is 1 mm. If the slit is illuminated by light of wavelenght 4800Å. The change in band width when the eyepiece is moved towards other the slit by 50 cm without distrubing the other arrangement is

In a Young's double slit experiment, the fringe width is found to be 0.4 m m . If the whole apparatus is immersed in water of refractive index 4 / 3 without disturbing the geometrical arrangement, the new fringe width will be

NIKITA PUBLICATION - INTERFERENCE AND DIFFRACTION - MULTPLE CHOICE QUESTIONS

In a biprism experiment, the wavelenght of monochromatic light is 6000...

In bipism experiment, the distance of 20^(th) bright band from the cen...

In a biprism experiment, fringe width is 0.4 mm when the eypiece is a...

In a biprism experimentn the the band width is found to be increased b...

In a biprism experimentn the distance between second and eight dark fr...

In a biprism experimentn the distance between the two virtual images ...

In a biprism experimentn the distancce virtua images of the slit is 2...

In a biprism experimentn is performed yellow light of wavelength 5600Å...

In a biprism experimentn the distance between slite and epepiece is 1m...

In a biprism experimentn the distance between the two virtual images o...

Two sources of light 0.85 mm apart are illuminated by a light of wavel...

The phenomenon of diffraction of light was discovered by-

Which of the following undergoe maximum diffraction ?

Phenomenon of bending of waves withoug a changed in medium is called

The penetration of light into the region of geometrical shadow is call...

For obtaining diffraction pattern, aperture of the slite should be of ...

A small circular disce is placed in the path of monochromatic light. T...

A very samml opaque is place in the path of monochormatic light. Its ...

The ratio of intensities of consecutive maxima in the diffraction patt...

A zone plae acts similar to a

Numerical Problems on Change in Fringe Width

• Post author By Hemant More
• Post date January 20, 2020
• No Comments on Numerical Problems on Change in Fringe Width

Science > Physics > Interference of Light > Numerical Problems on Fringe Width

In this article, we shall study numerical problems based on Young’s experiment and biprism experiment to find the fringe width of the interference pattern and to find the wavelength of light used.

Example – 12:

In a biprism experiment, the slit is illuminated by the light of wavelength 5890 Å . The distance between the slit and the eye-piece is 0.8 m. The two virtual images of the slit are formed 0.2 cm apart. Calculate the change in fringe width if the eye-piece is displaced 10 cm away from the slit.

Given: Distance between slits = d = 0.2 cm = 0.2 x 10 -2 m = 2 x 10 -3 m. Distance between slit and screen = D = 0.8 m, Wavelength of light = λ = 5890 Å = 5890 x 10 -10 m = 5.89 x 10 -7 m

To Find: Fringe width = X =?

The fringe width is given by X = λD/d

X = λD/d = (5.89 x 10 -7 x 0.8)/( 2 x 10 -3 ) = 2.356 x 10 -4 m = 0.2356 mm

Given: For first case: fringe width = X 1 = 0.2356 mm, distance of eye piece from the slits = D 1 = 0.8 m, For second case: distance of eye piece from the slits = D 2 = 0.8 m  + 10 cm = 0.8 m + 0.1 m = 0.9 m

To Find: Change fringe width = ∆X =?

For first case X 1 = λD 1 /d ………….. (1)

For second case, X 2 = λD 2 /d ………….. (2)

Dividing equation (2) by (1)

X 2 / X 1 = (λD 2 /d) x (d/ λD 1 )

∴ X 2 / X 1 = D 2 /D 1

∴ X 2 = (D 2 /D 1 ) x X 1

∴ X 2 = (0.9/0.8) x 0.2356 = 0.2651 mm

∴ Change in fringe width = ∆X = X 2 – X 1 = 0.2651 – 0.2356 = 0.0295 mm

Ans: The change in fringe width is 0.0295 mm

Example – 13:

In Young’s experiment the distance between two consecutive bright bands produced on a screen placed at 1.5 m from the two slits is 6.5 mm. What would be the fringe width if the screen is brought towards the slit by 50 cm for the same setting?

Given: For first case: fringe width = X 1 = 6.5 mm, distance of screen from the slits = D 1 = 1.5 m, For second case: distance of screen from the slits = D 2 = 1.5 m  – 50 cm = 1.5 m + 0.5 m = 1 m

To Find: New fringe width = X =?

For the first case X 1 = λD 1 /d ………….. (1)

For the second case, X 2 = λD 2 /d ………….. (2)

X 2 / X 1 = D 2 /D 1

X 2 = (D 2 /D 1 ) x X 1

X 2 = (1/1.5) x 6.5 = 4.33 mm

Ans: New fringe width is 4.33 mm

Example – 14:

In a biprism experiment, the fringe width is 0.4 mm when the eye-piece is at a distance of one metre from the slit. If now only the eye-piece is moved 25 cm towards the biprism, what would be the change in fringe width?

Given: For first case: fringe width = X 1 = 0.4 mm, distance of eye piece from the slits = D 1 = 1 m, for second case: distance of eye piece from the slits = D 2 = 1 m – 25 cm = 1 m + 0.25 m = 0.75 m

For first case  X 1 = λD 1 /d ………….. (1)

X 2 = (0.75/1) x 0.4 = 0.3 mm

Change in fringe width = ∆X = X 2 – X 1 = 0.4 – 0.3 = 0.1 mm

Ans: There is decrease of 0.1 mm in fringe width

Example – 15:

In biprism experiment, light of wavelength 5200 Å is used to get an interference pattern on a screen. The fringe width changes by 1.3 mm when the screen is brought towards the biprism by 50 cm. Find the distance between the virtual images of the slit.

Given: Wavelength of light used = λ = 5200 Å = 5200 x 10 -10 m = 5.2 x 10 -7 m, change in fringe width = ΔX = 1.3 mm = 1.3 x 10 -3 m, Initial distance of screen from virtual image = D 1 = D m, final distance of screen from virtual image = D 2 = D m – 50 cm = (D – 0.5) m

To Find:  distance between the virtual images of the slit = d =?

∆X = X 1 – X 2 = λD 1 /d – λD 2 /d = (λ/d)(D 1 – D 2 ) = (λ/d)(D – (D – 0.5)) =(λ/d)(0.5)

The change in fringe width = ∆X = (λ/2d)

d = (λ/2∆X) = (5.2 x 10 -7 ) / (2 x1.3 x 10 -3 ) = 2 x 10 -4 m = 0.2 mm

Ans: The distance between the virtual images of the slit is 0.2 mm

Example- 16:

In biprism experiment, the separation of the slits is halved and the distance between the slits and the screen is doubled. How is the fringe width affected?

Given: Distance between slits d 2 = ½ d 1 , Distance between screen and slit D 2 = 2 D 1

To Find: Change in fringe width = ∆X =?

For first case X 1 = λD 1 /d 1 ………….. (1)

For second case, X 2 = λD 2 /d 2 ………….. (2)

X 2 / X 1 = (λD 2 /d 2 ) x (d 1 / λD 1 )

X 2 / X 1 = (D 2 d 1 )/( D 1 d 2 )

X 2 / X 1 = (D 2 /D 1 ) x (d 1 /d 2 )

X 2 / X 1 = (2D 1 /D 1 ) x (d 1 /1/2 d 1 ) = 2 x 2 = 4

X 2 = 4 X 1

Ans: The fringe width increased 4 times

Example – 17:

In biprism experiment, interference fringes are observed at a distance of 60 cm from slits illuminated by monochromatic beam of light of wavelength 5460 Å . The distance between the slits is 3 mm. Find the change in fringe width if the distance between the slits is i) increased and ii) decreased by 1 mm.

Given: Distance between screen and sources = 60 cm = 0.6 m, Wavelength of light used = λ = 5460  Å = 5460 x 10 -10 m = 5.46 x 10 -7 m, Distance between slis = d = 3 mm = 3 x 10 -3 m.

Initial Fringe width = X = λD/d = (5.46 x 10 -7 x 0.6) / ( 3 x 10 -3 ) = 1.092 x 10 -4 m = 0.1092 mm

Part – I: The distance between slits is increased by 1 mm

Given: Initial fringe width = X 1 = 0.1092 mm, Initial distance between slits = d 1 = 3 mm, New distance between slits = d 2 = 3 + 1 = 4mm

To Find: the change in fringe width = ∆X =?

X 2 / X 1 = d 1 /d 2

X 2 = (d 1 /d 2 ) x X 1

X 2 = (3/4) x 0.1092 = 0.089

∆X = X 1 – X 2 =  0.1092 – 0.089 = 0.0273 mm

Part – Ii: The distance between slits is decreased by 1 mm

Given: Initial fringe width = X 1 = 0.1092 mm, Initial distance between slits = d 1 = 3 mm, New distance between slits = d 2 = 3 – 1 = 2mm

For first case X 1 = λD 1 /d 1 ………….. (1)

X 2 = (3/2) x 0.1092 = 0.1638

∆X = X 2 – X 1 =  0.1683 – 0.1092 = 0.0546 mm

Ans: When the distance between slits is increased by 1 mm, change in fringe width is 0.0273 mm. When the distance between slits is decreased by 1 mm, change in fringe width is 0.0546 mm

Example – 18:

In a biprism experiment, the distance of the slits from the screen is increased by 10% and the separation of slits is decreased by 20%. Find the percentage change in fringe width.

Given: Initial distance between the screen and slits = D 1 , New distance of screen from slits = D 2 = D 1 + 10%D 1 = 1.10 D 1 . Initial distance between the slits = d 1 , New distance between the slits = d 2 = d 1 – 20%d 1 = 0.80 d 1

X 2 / X 1 = (1.10 D 1 /D 1 ) x (d 1 /0.80d 1 )

X 2  = (1.10/0.80) x X 1

X 2  = 1.375 X 1

Change in fringe width = ∆X = 1.375X 1 – X 1 = 0.375 X 1

% change in fringe width = (∆X/X 1 ) x 100 = (0.375 X 1 /X 1 ) x 100 = 37.5

Ans: There is increase of 37.5 % in the fringe width

Example – 19:

In a biprism experiment, interference bands are observed at a distance of one metre from the slit. A convex lens put between the slit and the eye-piece gibes two images of slit 0.7 cm apart, the lens being 30 cm from the slit. Calculate the width of 10 bands if a light of wavelength 5892 Å is used.

Given: Wavelength of light used = 5892 Å = = 5892 x 10 -10 m = 5.892 x 10 -7 m, Distance between the screen and slit = D = 1m

To Find: Width of 10 bands = 10X =?

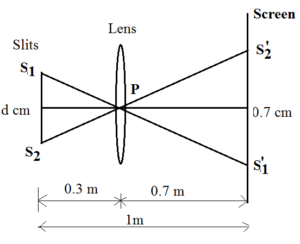
ΔS 1 ‘S 2 ‘ P and S 1 S 2 P are similar

d/0.3 = 0.7/0.7

d = 0.3 cm = 0.3 x 10 -3 m = 3 x 10 -4 m

Fringe width = X = λD/d = (5.892 x 10 -7 x 1)/( 3 x 10 -4 ) = 1.964 x 10 -4 m = 0.1964 mm

Width of 10 bands = 10 x 0.1964 = 1.964 mm

Ans: The width 10 bands is 1.964 m

Example – 20:

In a biprism experiment, a certain fringe width is observed when the green light of wavelength 5350 Å is used. The distance between the slit and the screen is 1.28 m. What should be the distance between the slit and the screen if the red light of wavelength 6400 Å. is used to get the same fringe width without disturbing the distance between the coherent sources?

Given: Initial wavelength = λ 1 = 5350 Å, Initial distance between the slit and screen = D 1 = 1.28 m, New wavelength = λ 2  = 6400 Å,

To Find: New distance between the slit and screen = D 2 =?

For first case X 1 = λ 1 D 1 /d ………….. (1)

For second case, X 2 = λ 2 D 2 /d ………….. (2)

Given X 1 = X 2

λ 1 D 1 /d= λ 2 D 2 /d

λ 1 D 1  = λ 2 D 2

D 2 = (λ 1 /λ 2 ) x D 1

D 2 = (5350/6400) x 1.28 = 1.07 m

Ans:  The distance between the slit and the screen for the red light is 1.07 m

Example – 21:

In biprism experiment when the slit is illuminated by light of wavelength 5890 Å, twenty fringes occupied 2.3 cm on the screen. When this light is substituted by another monochromatic light, thirty fringes occupied 2.8 cm on the screen. Find the wavelength of this light.

Given: Initial wavelength = λ 1 = 5890 Å, Initial fringe width = X 1 = 2.3/20 cm, New fringe width = X 2 = 2.8/30 cm,

To Find: New wavelength = λ 2 =?

For first case X 1 = λ 1 D/d ………….. (1)

For second case, X 2 = λ 2 D/d ………….. (2)

X 2 / X 1 = (λ 2 D/d) x (d/ λ 1 D)

X 2 / X 1 = λ 2 /λ 1

λ 2 = (X 2 /X 1 ) x λ 1  = ((2.8/30)/(2.3/20)) x 5890

λ 2 = (X 2 /X 1 ) x λ 1  = (5.6/6.9) x 5890 = 4780 Å

Ans: The new wavelength used is 4780 Å

Example – 22:

In a biprism experiment, fringes were obtained with a monochromatic source of light. The eye-piece was kept at a distance of 1 m from the slit and the bandwidth was measured. When another monochromatic source was used without disturbing the arrangement, the same bandwidth was obtained when the eye-piece was at 80 cm from the slit. Calculate the ratio of wavelengths of light from the two sources.

Given:  Initial distance between the slit and screen = D 1 = 1 m, New distance between the slit and screen = D 2 = 80 cm = 0.8 m

To Find: Ratio of wavelengths = λ 1 /λ 2 =?

For first case X 1 = λ 1 D 1 /d ………….. (1)

For second case, X 2 = λ 2 D 2 /d ………….. (2)

λ 1 /λ 2  = D 2 /D 1   = 0.8/1 = 8/10 = 4/5

Ans: The ratio of wavelengths used is 4:5

Previous Topic: More Problems on Fringe Width and Change of Fringe Width

For More Topics in Physics Click Here

• Tags Bandwidth , Bright band , Bright fringe , Bright point , Coherent sources , Constructive interference , Dark band , Dark fringe , Dark point , Destructive interference , Fresnel's biprism experiment , Fringe width , in phase , Interference of light , Maxima , Minima , Monochromatic light , out of phase , Path difference , Principle of superposition of waves , Steady interference pattern , Wavelength of light , Young's double-slit experiment

Leave a Reply Cancel reply

Your email address will not be published. Required fields are marked *

In a biprism experiment, fringe width is 0.4 mm when the eyepiece is at a distance of 1 m from the slit. If the eyepiece is moved through a distance of 25 cm towards biprism without changing any other arrangement then the change in fringe width is 0.1 mm 0.2 mm 0.15 mm 0.25 mm

X = d λ d 0.4 = 1 × λ d ⟶ 1 x = 0.75 λ d ⟶ 2 1 − 0.25 = 0.75 e q u a t i o n 1 e q u a t i o n 2 ⇒ 0.4 x = 1 3 4 = 4 3 x = 3 × 0.4 4 = 0.3 difference in fringe width = 0.4 − 0.3 = 0.1 mm.